Cycle I Advanced Placement Physics B
John Dewey High School
Mr. Klimetz
Gravitation and Planetary Motion: Calculating the Kepler Constant for a System of Orbiting Bodies (Natural and Artificial)
Evaluating the Relationships Between Newton's Laws of Motion and the Universal Law of Gravitation and Their Application in Explaining the Physics of Planets, Satellites, and Any System of Orbiting Bodies
Laboratory Exercise No. 6
Introduction
How did Newton's Laws of Motion lead to the development of the Universal Law of Gravitation?
How can we demonstrate that the Universal Law of Gravitation applies to all matter in the Universe?
How can we demonstrate the application of the Universal Law of Gravitation to explain motion of planets,
satellites, and orbiting bodies?

Newton's Second Law Applied to Astronomy. One of the great triumphs of the Law of Acceleration, that is, force equals the product of mass and acceleration (F = ma), was its success in explaining precisely the motion of the planets in their orbits around the Sun. This required knowing what forces were acting on the planets and thus controlling their motion. Newton provided that information when he derived the Universal Law of Gravitation. Using this law in conjunction with the Law of Acceleration and its variants, it then became possible to correctly and precisely compute the motions not only of the planets, but also of the Moon, comets, and other celestial bodies. Today, Newton's discoveries and methods continue to be applied with enormous success in the launching of satellites and space vehicles.
Problem of Gravitation. Specifically, the problems that Newton was trying to solve, and those with which we are concerned in this particular laboratory exercise, are the following:
What is the true nature of the force that accelerates all objects towards Earth's center and how can this be quantitatively expressed?
What is the nature of the force exerted by the Sun that gives the planets the centripetal acceleration needed to keep them in their orbits and how can it be quantitatively expressed?
How can the observed relationship between the orbital radius, orbital speed, and mass of natural satellites in our solar system be applied to the launching of artificial satellites as well as to the motions of bodies in hypothetical solar systems?
How does the strength of an object's gravitational field change with increasing distance from its center and how can this be quantitatively expressed?
Objectives
In this particular exercise you are going to evaluate the dynamic and kinematic relationships between three orbiting members of a hypothetical solar system and their central star. On the basis of your understanding of two-dimensional kinematics, Newton's Laws of Motion, the Universal Law of Gravitation, Kepler's Third Law of Planetary Motion, centripetal acceleration, and centripetal force, you are expected to
Calculate the force of attraction (Fg) between each planet and its central star.
Calculate the field strength (g) of the central star at each planet.
Calculate the average orbital velocity (v) for each planet.
Calculate the average orbital period (T) of each planet.
Calculate the orbital circumference (C) of each planet.
Confirm Kepler's Third Law by calculating the Kepler Constant (k) for each planet
Materials
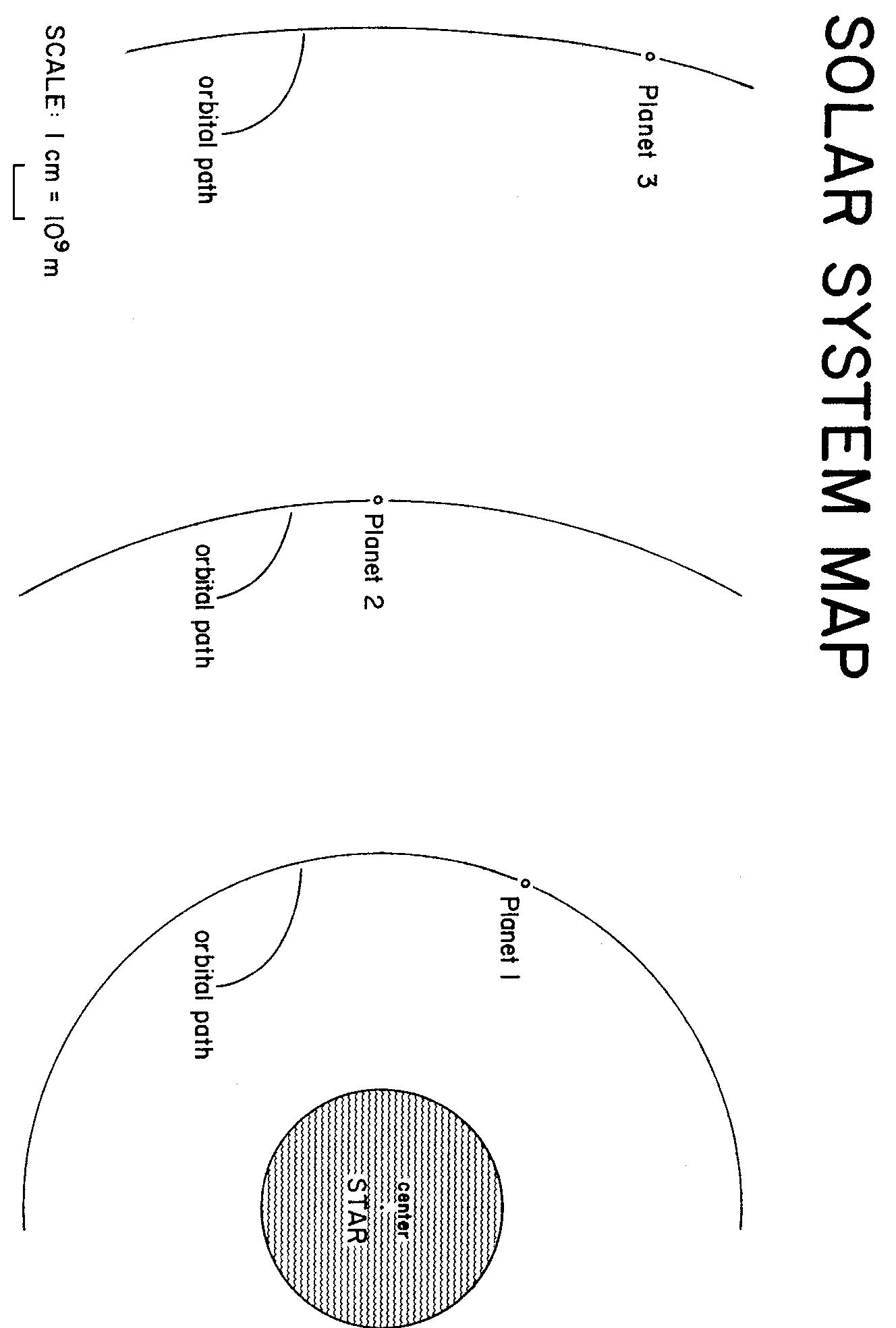
Solar System Map showing positions of central star, planets, and orbits
"Nerf" foam plastic model of central star (half of a 4.50 cm diameter sphere)
Glass/polystyrene single-hole bead models of planets (three-0.30 cm-diameter spheres)
Four thumbtacks
Metric ruler
Scientific calculator
Graph paper
Pencils with erasers
Procedure
1.   Assemble a single model of the solar system using the foam "Nerf" ball and glass/plastic beads provided from a separate Solar System Map sheet for the entire lab for reference purposes. Thumbtacks can be inserted through the bottom of the sheet so that their protruding points can serve as mounts for the planets and central star. Each lab member must have their own Solar System Map sheet. Caveat: Be mindful of the fact that this map sheet has been drawn to-scale and that all of the planets lie in the same plane which in turn bisects the central star.
2.   Measure all required distances such as stellar and planetary radii, orbital radii, etc., directly from the Solar System Map and model sheets and record in the places provided. (Map Scale: 1 cm = 10exp9 m)
3.  Perform all calculations and graph all data sets as instructed.
4.  Answer any follow-up questions provided by your instructor at the end of your report.
Data
Radii of Celestial Bodies
Radius of Central Star (rs) = 2.25 cm = ________ m
Radius of Planet 1 (r1) = 0.15 cm = ________ m
Radius of Planet 2 (r2) = 0.15 cm = ________ m
Radius of Planet 3 (r3) = 0.15 cm = ________ m
Orbital Radii of Planets
Orbital Radius of Planet 1 (R1) = ________ cm = ________ m
Orbital Radius of Planet 2 (R2) = ________ cm = ________ m
Orbital Radius of Planet 3 (R3) = ________ cm = ________ m
Masses of Celestial Bodies
Mass of Central Star (ms) = 1.6 x 10exp31 kg
Mass of Planet 1 (m1) = 9.8 x 10exp28 kg
Mass of Planet 2 (m2) = 8.4 x 10exp28 kg
Mass of Planet 3 (m3) = 7.0 x 10exp28 kg
Calculations
A. Calculate the force of gravitational attraction (Fg) between each planet and the central star.
Fg1 = ________ N
Fg2 = ________ N
Fg3 = ________ N
B. Calculate the gravitational field strength (g) of the central star at each planet.
g1 = ________ m/sexp2
g2 = ________ m/sexp2
g3 = ________ m/sexp2
C. Calculate the average orbital velocity (v) of each planet.
v1 = ________ m/s
v2 = ________ m/s
v3 = ________ m/s
D. Calculate the orbital circumference (C) of each planet.
C1 = ________ m
C2 = ________ m
C3 = ________ m
E. Calculate the orbital period (T) of each planet.
T1 = ________ s
T2 = ________ s
T3 = ________ s
F. Calculate the Kepler Constant (k) of each planet.
k1 = ________ mexp3/sexp2
k2 = ________ mexp3/sexp2
k3 = ________ mexp3/sexp2
Graphing
A.   Plot Fg (N) versus R (m) for each planet. Gravitational force (Fg) should appear on the ordinate and orbital radius (R) should appear on the abscissa. Use different colored dots to indicate each planet. Connect with a smooth curve.
B.   Plot g (m/sexp2) of the central star versus R (m) at the location of each planet. Gravitational field strength (g) should appear on the ordinate and orbital radius (R) should appear on the abscissa. Use different colored dots to indicate each planet. Connect with a smooth curve.
C.   Plot v (m/s) versus R (m) for each planet. Average orbital velocity (v) should appear on the ordinate and orbital radius (R) should appear on the abscissa. Use different colored dots to indicate each planet. Connect with a smooth curve.
Problems
A.   Based on your understanding of the Universal Law of Gravitation and the weight equation,
calculate the local gravitational acceleration at the surface of the star.
B.   Based on your understanding of the Universal Law of Gravitation and the weight equation,
calculate the weight of a 5.00 kg mass at the surface of the star.
C.   Based on your understanding of the Universal Law of Gravitation and the centripetal force equation, calculate the minimum speed required to launch a satellite into an orbit around the star at its surface.
D.   Based on your understanding of the Universal Law of Gravitation, the centripetal force equation, and your calculation of the Kepler Constant [k] for the given solar system, calculate the orbital radius at which the orbital period of a satellite would be exactly one year.
E.   Based on your understanding of the Universal Law of Gravitation, the centripetal force equation, and your calculation of the Kepler Constant [k] for the given solar system, calculate the orbital period of a satellite located at an average orbital radius of 4.50 x 10exp9 m.